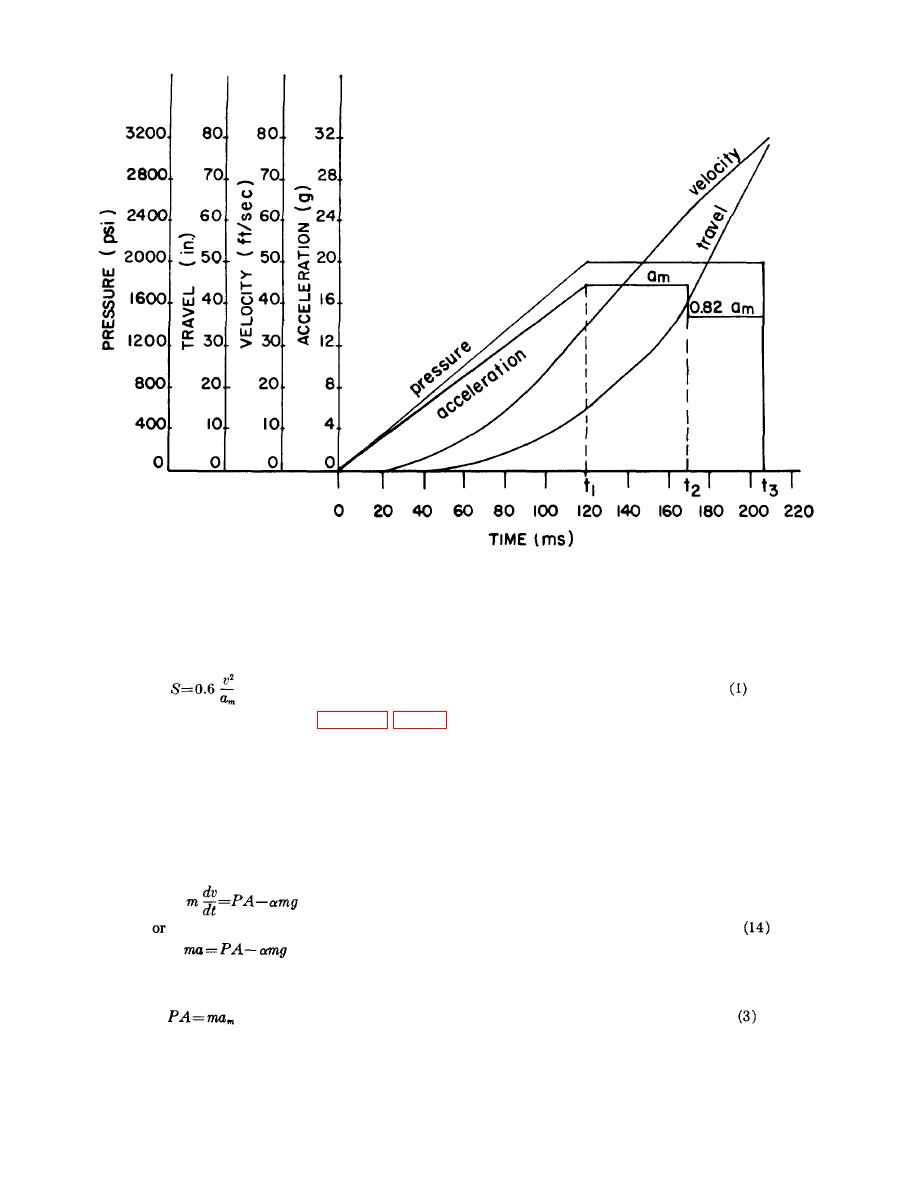
Figure 46. "Ideal" pressure, acceleration, velocity, and travel vs time curves for a three-tube catapult.
2
3
The second term may be eliminated, since the value of 24 is so large that it makes the term am
insignificant. Firing tests have indicated that for a closer approximation of stroke length, equation
(27), with the elimination of the second term, should be modified by increasing the values by 20
percent. The equation then becomes
which is plotted in chapter 4 (fig. 20) showing stroke length as a function of terminal velocity for
several values of maximum acceleration.
(g) It
should be
noted that these parameters (acceleration, velocity, and travel) are independent of
the
mass to be accelerated. The mass fixes only the required pressure and tube areas as will be shown
in b below.
b. Pressure and Tube Area Relationships.
(1) The pressure and tube area relationship is based upon the equation of motion
Substituting am for a and eliminating αmg (since αamg <<PA), the equation relating piston area to peak
pressure becomes:
53