acceleration limit and, therefore, the peak pressure at the high temperature extreme. Operation at a lower
temperature will result in a lower peak pressure. The peak pressure in a propellant actuated device may
be varied by changing the propellant web, the propellant composition, or the web and composition
simultaneously. A reduction in web or use of a higher burning rate propellant will increase the peak
pressure; conversely, an increase in web thickness or use of a lower burning rate propellant will decrease
the peak pressure. Use of a plateau propellant may also lower the peak pressure if a propellant is chosen
which has a plateau at the desired operating pressure. If a change in metal parts is permitted, the internal
volume and piston area may be increased to lower the peak pressure. A change in peak pressure may
affect the rate of change of acceleration. Generally, a higher peak pressure will cause a higher rate of
change of acceleration, while a reduction in the peak pressure will cause a lower rate of change of
acceleration.
(4) Integral of the pressure vs time curve.
(a) The integral of the pressure-time curve is proportional to the terminal velocity. If the terminal
velocity is too low, the propellant charge weight must be increased. It is desirable for the P-t curve to
be flat (a slight increase in pressure toward the end of the cycle is desirable in catapult applications if
telescoping tubes are used with a reduction in tube area) so that for a given stroke length the
terminal velocity will be higher. A drop (negative slope) in the pressure-time (P-t) curve may be
lessened or eliminated by use of a more progressive grain design in which the surface-time
relationship increases faster as the grains burn. Several grain designs mentioned in the preceding
paragraph will provide a progressive surface-time history.
(b) The propellant web should also be sized so that burnout occurs at or slightly after tube separation.
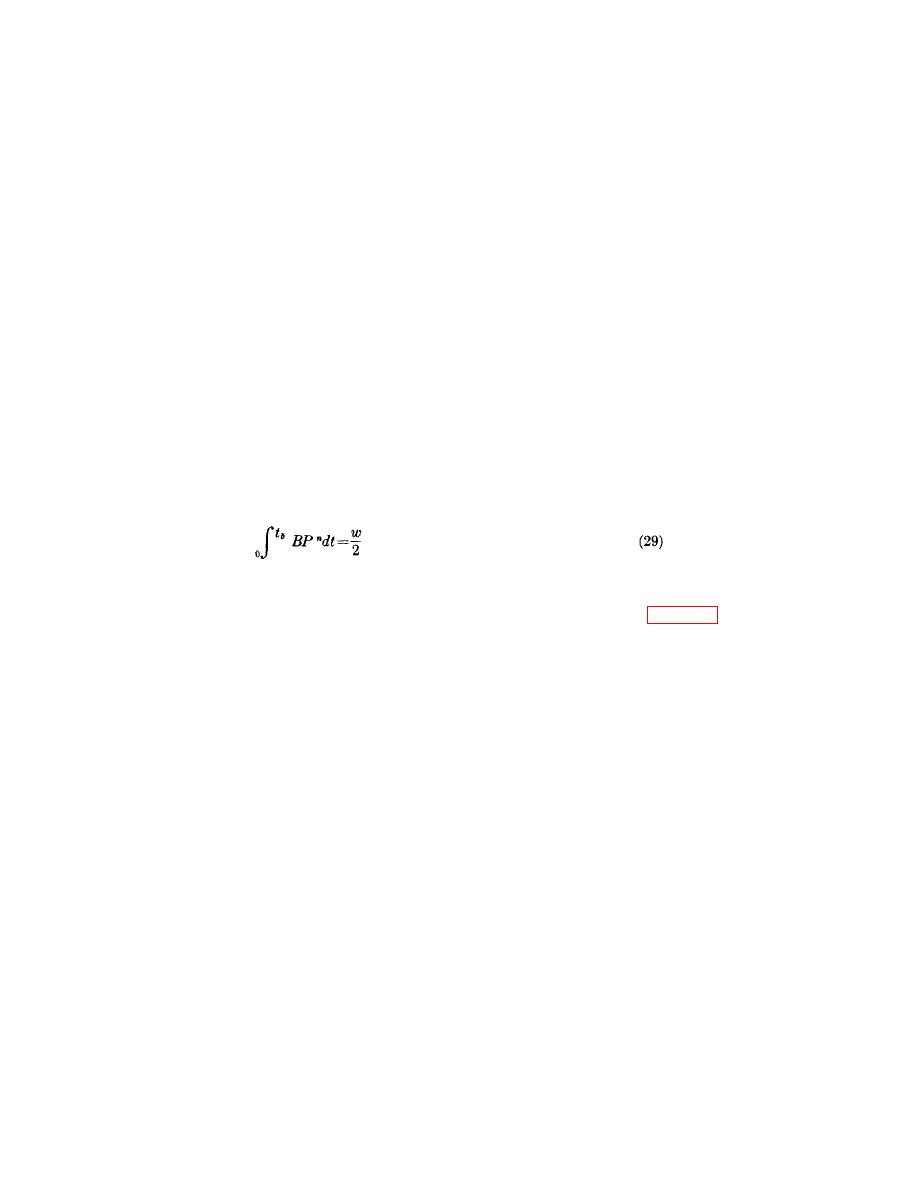
(c) With a given pressure-time curve and the equations describing the burning process, the time at
which propellant burning has ceased can be calculated. The time at which the web has burned out
n
n
can be found by replotting the pressure-time curve as BP , vs time. Integrations of the curve of BP
vs time over the interval t = 0 to t = tb should be made to satisfy the equation
The upper limit of the integral, to, represents the time of all burnt.
(d) In an
experiment in
which propellant combustion is
completed during the burning cycle, the end of
burning can be determined by preparing a graph such as shown in figure 48. After gas production
a
has ceased, the pressure and volume are related functionally by the polytropic law (Pv = constant).
On logarithmic scales, this function is a straight line. The point of tangency of the straight line with
the curve corresponds to burnout of the propellant.
Section II. MATHEMATICAL ANALYSIS OF INTERIOR BALLISTICS
53. Catapults and Removers. a. A mathematical description of ballistic performance consists of a group of
simultaneous equations with time as the independent variable. These equations, which describe the conservation of
energy, the motion of the propelled mass, and the production of propellant gas, form the basis of any theory of interior
ballistics.
b. The following assumptions usually are made in an analysis of the interior ballistics of propellant actuated
devices:
(1) Ignition is isochronic; that is, stable combustion is established over the entire propellant surface at the
beginning of the ballistic cycle.
(2) The rate of production of propellant gas is determined by the geometry of the grain and the burning rate. It
is assumed that all dimensions of the propellant grain recede in a direction normal to all uninhibited
surfaces at the same rate.
60